Calculus on Manifolds
Math 4B03/6B03, Winter 2012
Dr. Ben Mares
$$\int_M d\omega = \int_{\partial M} \omega.$$
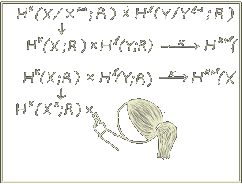
(Credit: Abstruse Goose)

Announcements
- Doodle link for extra optional lecture, location TBA
- Some of you may be interested in the undergraduate summer school at Notre Dame. See the link at the bottom of the page.
- I always appreciate constructive feedback via my anonymous survey.
- If you get stuck, please feel free to come to office hours and/or discuss with your classmates!
- Office hours: Tu Th 1-2pm, or by appointment.


Final evaluation
- Congrats to everyone who has completed their final evaluation!
- A single file containing all the problems for the final evaluation
- Here is the spreadsheet listing the problems.
Readings
This is suggested reading for the upcoming lectures. Be warned that I sometimes change my mind, but I will do my best to keep this up-to-date.Tuesday, Apr 3
Topics: Cohomology of $S^n$ using Mayer-Vietoris, Outline of Mayer-Vietoris, closing perspectives
References: M&T Chapters 4&5
Homework
- Due at the beginning of class or earlier.
- Problem Set #11 due Tuesday, April 3rd. (Last one!)
- Problem Set #10 due Friday, March 23rd.
- Problem Set #9 due Friday, March 16th.
- Problem Set #8 due Friday, March 9th.
- Problem Set #7 due Tuesday, March 6th.
- Problem Set #6 due Friday, February 17th.
- Problem Set #5 due Friday, February 10th.
- Problem Set #4 due Friday, February 3rd.
- Problem Set #3 due Friday, January 27th.
- Problem Set #2 due Friday, January 20th.
- Problem Set #1 due Thursday, January 12th.
Notes
-
Me:
- Last lecture
- Manifolds don't retract to their boundary
- Lecture on Stokes' Theorem
- Visualizing orientations
- Transformation of tangent and cotangent vectors
- The Poincaré lemma and homotopy invariance of cohomology
- Lecture notes about $\otimes$
- Supplementary notes for §1
- Glossary
- Differentiable Manifolds by Nigel Hitchin The Poincaré lemma and de Rham Cohomology by Daniel Litt
- Boy's surface, a counterintuitive immersion of $\mathbb{RP}^2$ into $\mathbb{R}^3$.
- I wish I had used The Geometry of Physics as our textbook. This is an excellent reference for most of the material we covered. It is extremely broad.
- Differential Forms in Algebraic Topology is a masterpiece, and probably my favorite textbook ever. I'm proud to say that you are now prepared to tackle this, should you choose to journey deeper into algebraic topology.
Others:
Future:
The big picture
Where have we been?- Intro (M&T Chapter 1)
- Tensor algebra and tensor calculus (Hitchin Chapter 2, Section 5)
- Alternating algebra (M&T Chapter 2, excluding characteristic polynomials (2.16))
- de Rham cohomology and Poincaré lemma (M&T Chapter 3)
- Homotopy (M&T Chapter 6, only 6.7, 6.8, 6.10)
- Smooth manifolds (M&T Chapter 8)
- Differential forms on manifolds (M&T Chapter 9, excluding 9.16 and after).
- Integration on manifolds (M&T Chapter 10)
- Chain complexes and cohomology (M&T Chapter 4)
- Mayer-Vietoris sequence (M&T Chapter 5)
- On to do exciting new things!
More info
- Course Outline
- Introduction to topological invariants in low dimensions (Undergraduate Summer School at Notre Dame, May 21-26.)